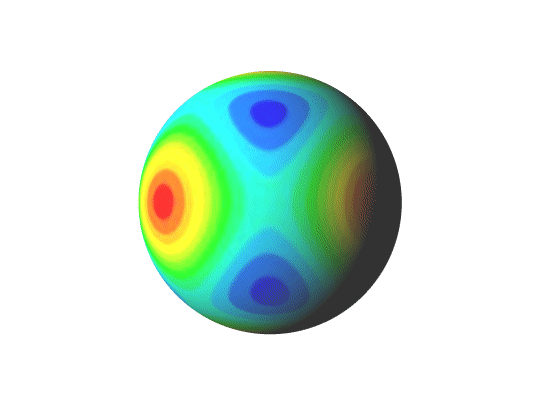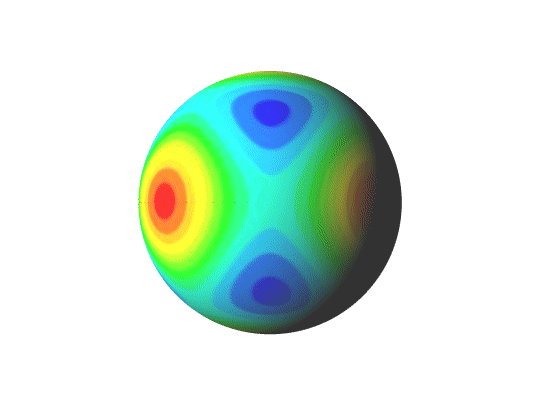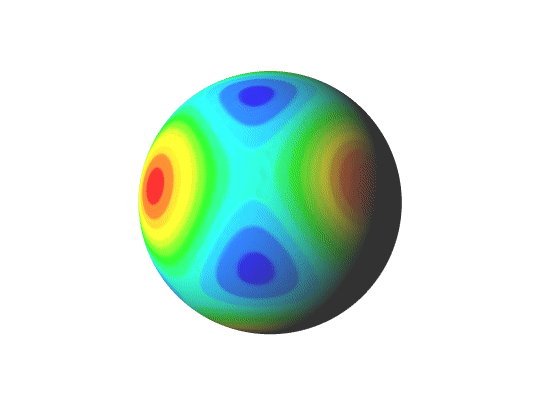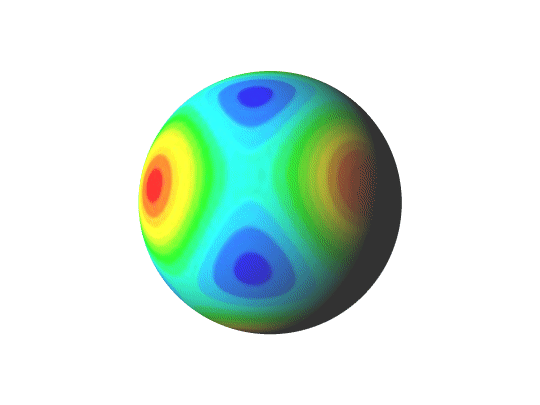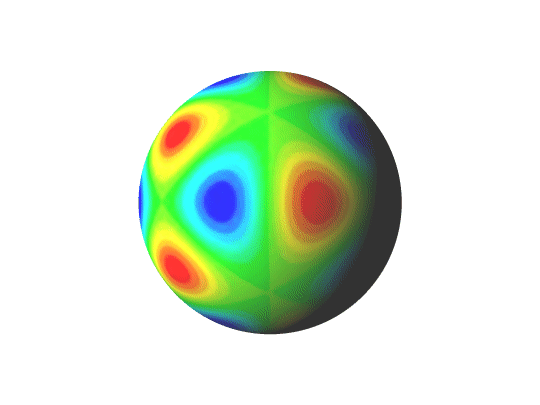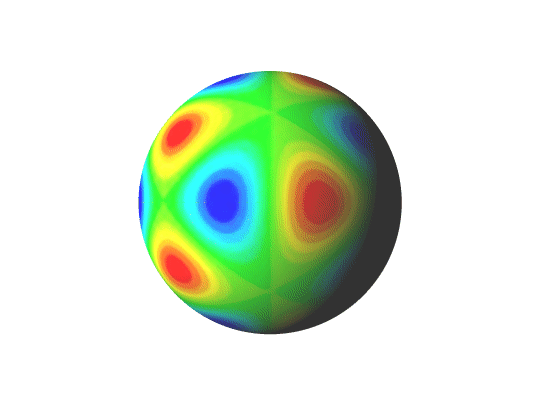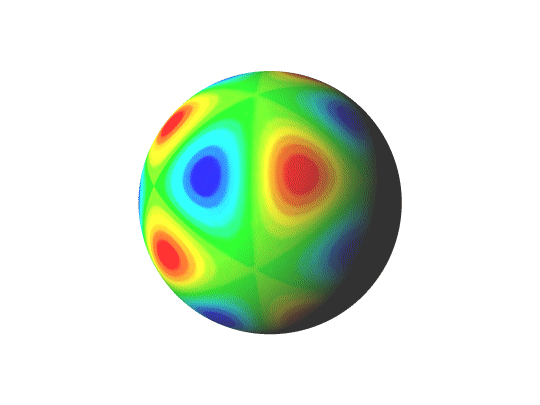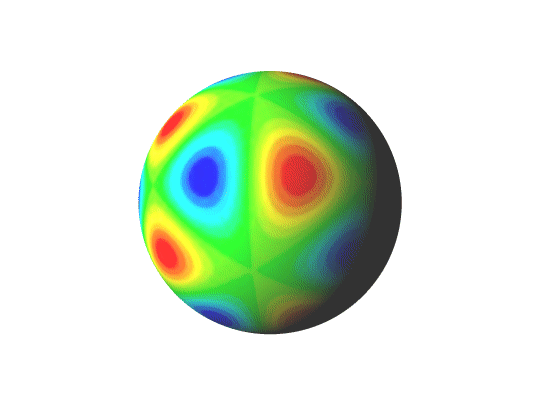正四面体型調和関数(その1)
正四面体群\(T_{d}\)の指標表と球面調和関数
正四面体群\(T_{d}\)の指標表は、
\[
\begin{array}{|c|c|c|c|c|c|}
\hline
& E & C_{2} & C_{3} & IC_{4} & \sigma \\
\hline
A_{1} & 1 & 1 & 1 & 1 & 1 \\
\hline
A_{2} & 1 & 1 & 1 & -1 & -1 \\
\hline
E & 2 & 2 & -1 & 0 & 0 \\
\hline
T_{1} & 3 & -1 & 0 & 1 & -1 \\
\hline
T_{2} & 3 & -1 & 0 & -1 & 1 \\
\hline
\end{array}
\]
一方、球面調和関数\(Y^{M}_{L}(\theta ,\phi)\)は、\(SO(3)\)の\((2L+1)\)次元既約表現\(D^{(L)}\)の基底で、\(O(3)\)の基底としては、空間反転\(I\)に対し、
\[IY^{M}_{L}(\theta,\phi)=Y^{M}_{L}(\pi-\theta,\phi+\pi )=(-1)^{L}Y^{M}_{L}(\theta,\phi)\]
と変換するので、\(O(3)\)を\(T_{d}\)に制限したときの\(D^{(L)}\)の指標\(\chi^{(L)}\)は、
\[\chi^{(L)}(E)=2L+1\]
\[\chi^{(L)}(C_{2})=(-1)^{L}\]
\[\chi^{(L)}(C_{3})=1 (L=0 \bmod 3), 0 (L=1 \bmod 3), -1 (L=2 \bmod 3)\]
\[\chi^{(L)}(IC_{4})=1 (L=0,3 \bmod 4), -1 (L=1,2 \bmod 4)\]
\[\chi^{(L)}(\sigma)=\chi^{(L)}(IC_{2})=1\]
であり、\(D^{(L)}\)を\(T_{d}\)の既約表現に分解したときの重複度は、
\[
\begin{array}{|c|c|c|c|c|c|}
\hline
L & 0 & 1 & 2 & 3 & 4 & 5 & 6 & 7 & 8 & 9 & 10 & 11 & 12 \\
\hline
A_{1} & 1 & 0 & 0 & 1 & 1 & 0 & 1 & 1 & 1 & 1 & 1 & 1 & 2 \\
\hline
A_{2} & 0 & 0 & 0 & 0 & 0 & 0 & 1 & 0 & 0 & 1 & 1 & 0 & 1 \\
\hline
E & 0 & 0 & 1 & 0 & 1 & 1 & 1 & 1 & 2 & 1 & 2 & 2 & 2 \\
\hline
T_{1} & 0 & 0 & 0 & 1 & 1 & 1 & 1 & 2 & 2 & 2 & 2 & 3 & 3 \\
\hline
T_{2} & 0 & 1 & 1 & 1 & 1 & 2 & 2 & 2 & 2 & 3 & 3 & 3 & 3 \\
\hline
\end{array}
\]
となる。\(C_{2}, C_{3}, IC_{4}\)の指標が\(L\)についてそれぞれ\(2, 3, 4\)の周期をもつので、合わせて\(12\)の周期となり、\(L=12\)以上では\(L-12\)との差が\(E\)からの寄与だけとなる。\(T_{d}\)の既約表現のうちで、\(A_{1}\)が、すべての元に対して不変となる表現で、\(A_{2}\)が、空間反転\(I\)を含む元について、符号のみが変わる表現となる。
\(L=3\)の場合
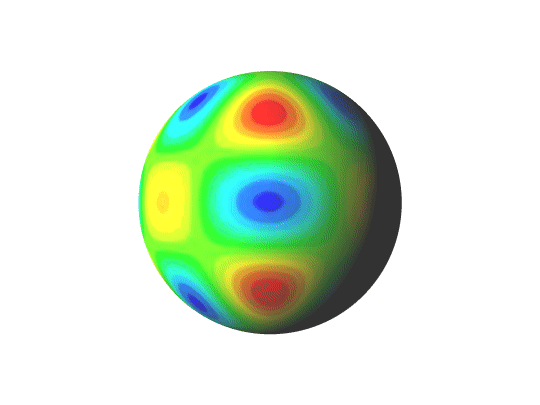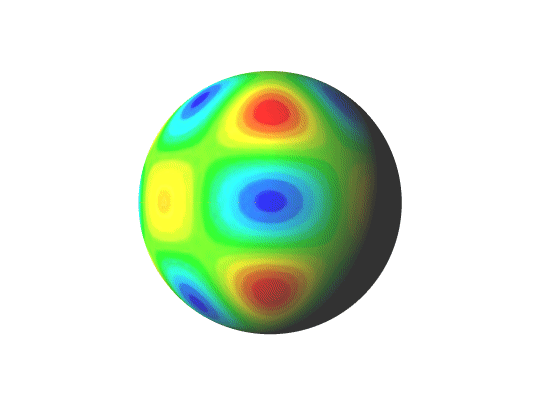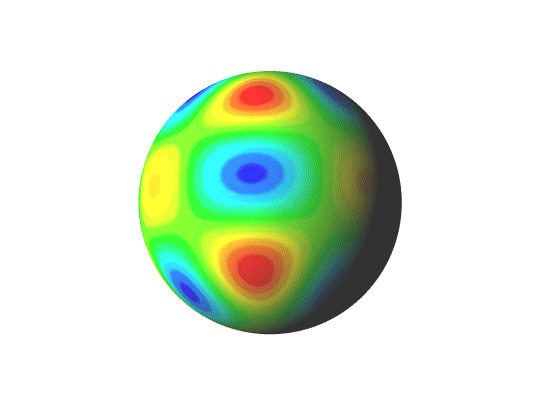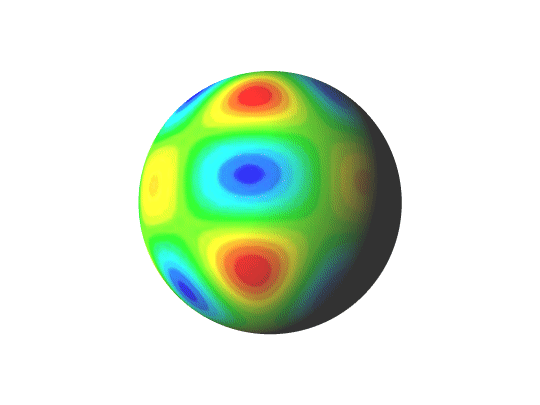
正四面体の\(C_{2}\)軸が\(z\)軸に一致すると仮定すると、成分が\(M=0 (\bmod 2)\)に限られるので、\(L=3\)で正四面体対称性をもつベクトル\(|\psi_{3}>\)は
\[|\psi_{3}>=a|3,2> + b|3,0> + c|3,-2>\]
で表される。さらに、他の\(C_{2}\)軸が\(y\)軸に一致すると仮定すると、\(y\)軸回りの\(1/2\)回転の表現行列は
\[D^{(L)}_{km} = (-1)^{L+k}\delta_{k,-m}\]
であるから、\(1/2\)回転で
\[a|3,2>+b|3,0>+c|3,-2> \ \to -a|3,-2>-b|3,0>-c|3,2>\]
と変換する。\(L=3\)の場合、\(1\)次元表現は\(A_{1}\)表現だけなので、これが不変であるためには、\(a=-c, b=0\)が必要となる。規格化条件から、\(L=3\)の場合の\(A_{1}\)表現の基底は、
\[|\psi_{3}>=\sqrt{\frac{1}{2}}(|3,2> - |3,-2>)\]
となる。
\(L=4, 6, 7\)の基底を取り出す
まず、\(2\)つの\(L=3\)の合成系は、
\[|\psi_{3}>|\psi_{3}>=\frac{1}{2}(|3,3,2,2>-|3,3,2,-2>-|3,3,-2,2>+|3,3,-2,-2>)\]
であるが、そのうちの\(L=4, 6\)の成分に注目すると、\(3j\)記号を用いて、
\begin{align*}
<4,4|3,3,2,2>=(-1)^{3-3+4}\sqrt{2\cdot 4+1}\begin{pmatrix} 3 & 3 & 4 \\ 2 & 2 & -4 \end{pmatrix},\
& \begin{pmatrix} 3 & 3 & 4 \\ 2 & 2 & -4 \end{pmatrix} =-\frac{1}{3}\sqrt{\frac{5}{11}} \\
<4,0|3,3,2,-2>=(-1)^{3-3+0}\sqrt{2\cdot 4+1}\begin{pmatrix} 3 & 3 & 4 \\ 2 & -2 & 0 \end{pmatrix},\
& \begin{pmatrix} 3 & 3 & 4 \\ 2 & -2 & 0 \end{pmatrix} =\frac{1}{3}\sqrt{\frac{7}{2\cdot 11}} \\
<6,4|3,3,2,2>=(-1)^{3-3+6}\sqrt{2\cdot 6+1}\begin{pmatrix} 3 & 3 & 6 \\ 2 & 2 & -4 \end{pmatrix},\
& \begin{pmatrix} 3 & 3 & 6 \\ 2 & 2 & -4 \end{pmatrix} =\sqrt{\frac{2\cdot 3}{11\cdot 13}} \\
<6,0|3,3,2,-2>=(-1)^{3-3+0}\sqrt{2\cdot 6+1}\begin{pmatrix} 3 & 3 & 6 \\ 2 & -2 & 0 \end{pmatrix},\
& \begin{pmatrix} 3 & 3 & 6 \\ 2 & -2 & 0 \end{pmatrix} =\sqrt{\frac{3}{7\cdot 11\cdot 13}} \\
\end{align*}
\[<4,-4|3,3,-2,-2>=(-1)^{3+3-4}<4,4|3,3,2,2>=<4,4|3,3,2,2>\]
\[<6,-4|3,3,-2,-2>=(-1)^{3+3-6}<6,4|3,3,2,2>=<6,4|3,3,2,2>\]
より、
\[|\psi_{4}>\propto -\frac{1}{6}\sqrt{\frac{5}{11}}(|4,4>+|4,-4>)-\frac{1}{3}\sqrt{\frac{7}{2\cdot 11}}|4,0>\]
\[|\psi_{6}>\propto \frac{1}{2}\sqrt{\frac{2\cdot 3}{11\cdot 13}}(|6,4>+|6,-4>)-\sqrt{\frac{3}{7\cdot 11\cdot 13}}|6,0>\]
なので、規格化条件から、
\[|\psi_{4}>=\frac{1}{12}\sqrt{2\cdot 3\cdot 5}(|4,4>+|4,-4>)+\frac{1}{6}\sqrt{3\cdot 7}|4,0>\]
\[|\psi_{6}>=\frac{1}{4}\sqrt{7}(|6,4>+|6,-4>)-\frac{1}{4}\sqrt{2}|6,0>\]
である。
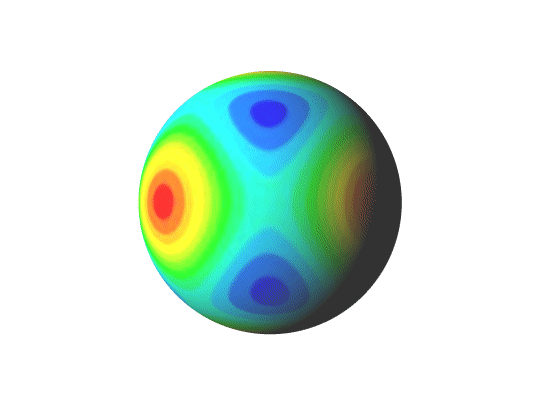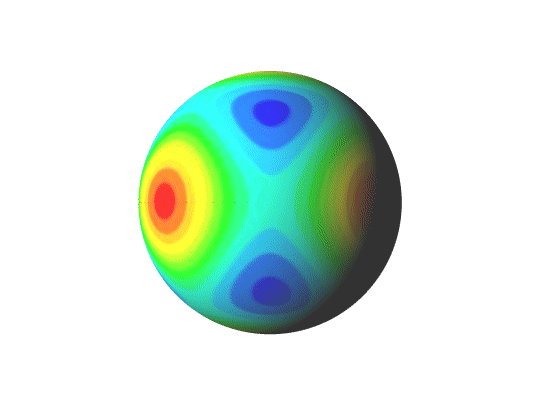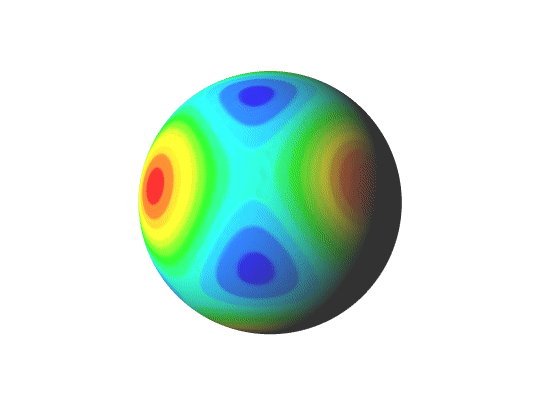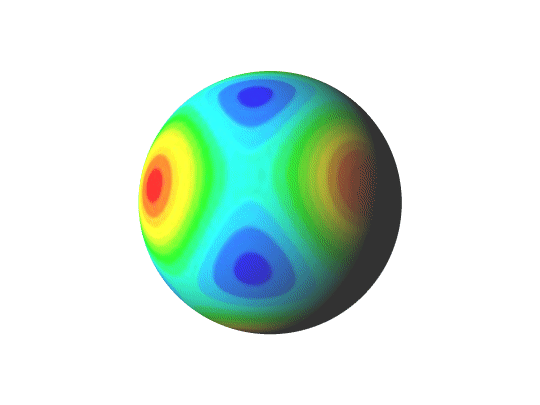
次に、\(L=3\)と\(L=4\)の合成系は、
\begin{align*}
|\psi_{3}>|\psi_{4}>&=\frac{1}{24}\sqrt{6}(|3,2> - |3,-2>)(\sqrt{5}(|4,4>+|4,-4>)+\sqrt{2\cdot 7}|4,0>) \\
&=\frac{1}{24}\sqrt{6}(\sqrt{5}(|3,4,2,4>-|3,4,-2,4>+|3,4,2,-4>-|3,4,-2,-4>) \\
& \ +\sqrt{2\cdot 7}(|3,4,2,0>-|3,4,-2,0>))
\end{align*}
であるが、そのうちの\(L=6, 7\)の成分に注目すると、\(3j\)記号を用いて、
\begin{align*}
<6,6|3,4,2,4>=(-1)^{3-4+6}\sqrt{2\cdot 6+1}\begin{pmatrix} 3 & 4 & 6 \\ 2 & 4 & -6 \end{pmatrix},\
& \begin{pmatrix} 3 & 4 & 6 \\ 2 & 4 & -6 \end{pmatrix} =2\sqrt{\frac{1}{7\cdot 13}} \\
<6,2|3,4,-2,4>=(-1)^{3-4+2}\sqrt{2\cdot 6+1}\begin{pmatrix} 3 & 4 & 6 \\ -2 & 4 & -2 \end{pmatrix},\
& \begin{pmatrix} 3 & 4 & 6 \\ -2 & 4 & -2 \end{pmatrix} =\frac{2}{3}\sqrt{\frac{5}{7\cdot 11\cdot 13}} \\
<6,2|3,4,2,0>=(-1)^{3-4+2}\sqrt{2\cdot 6+1}\begin{pmatrix} 3 & 4 & 6 \\ 2 & 0 & -2 \end{pmatrix},\
& \begin{pmatrix} 3 & 4 & 6 \\ 2 & 0 & -2 \end{pmatrix} =-\frac{4}{3}\sqrt{\frac{2}{11\cdot 13}} \\
<7,6|3,4,2,4>=(-1)^{3-4+6}\sqrt{2\cdot 7+1}\begin{pmatrix} 3 & 4 & 7 \\ 2 & 4 & -6 \end{pmatrix},\
& \begin{pmatrix} 3 & 4 & 7 \\ 2 & 4 & -6 \end{pmatrix} =-\sqrt{\frac{1}{5\cdot 7}} \\
<7,2|3,4,-2,4>=(-1)^{3-4+2}\sqrt{2\cdot 7+1}\begin{pmatrix} 3 & 4 & 7 \\ -2 & 4 & -2 \end{pmatrix},\
& \begin{pmatrix} 3 & 4 & 7 \\ -2 & 4 & -2 \end{pmatrix} =-\sqrt{\frac{1}{5\cdot 7\cdot 11\cdot 13}} \\
<7,2|3,4,2,0>=(-1)^{3-4+2}\sqrt{2\cdot 7+1}\begin{pmatrix} 3 & 4 & 7 \\ 2 & 0 & -2 \end{pmatrix},\
& \begin{pmatrix} 3 & 4 & 7 \\ 2 & 0 & -2 \end{pmatrix} =-\sqrt{\frac{2}{11\cdot 13}} \\
\end{align*}
\[<6,-2|3,4,-2,0>=(-1)^{3+4-6}<6,2|3,4,2,0>=-<6,2|3,4,2,0>\]
\[<6,-2|3,4,-2,-4>=(-1)^{3+4-6}<6,2|3,4,-2,4>=-<6,2|3,4,-2,4>\]
\[<6,-6|3,4,-2,-4>=(-1)^{3+4-6}<6,6|3,4,2,4>=-<6,4|3,4,2,4>\]
\[<7,-2|3,4,-2,0>=(-1)^{3+4-7}<7,2|3,4,2,0>=<7,2|3,4,2,0>\]
\[<7,-2|3,4,-2,-4>=(-1)^{3+4-7}<7,2|3,4,-2,4>=<7,2|3,4,-2,4>\]
\[<7,-6|3,4,-2,-4>=(-1)^{3+4-7}<7,6|3,4,2,4>=<7,4|3,4,2,4>\]
より、
\[|\psi_{6}>\propto 2\sqrt{\frac{5}{7\cdot 13}}(|6,6>+|6,-6>)-22\sqrt{\frac{1}{7\cdot 11\cdot 13}}(|6,2>+|6,-2>)\]
\[|\psi_{7}>\propto \sqrt{\frac{1}{7}}(|7,6>-|7,-6>)-13\sqrt{\frac{1}{7\cdot 11\cdot 13}}(|7,2>-|7,-2>)\]
なので、規格化条件から、
\[|\psi_{6}>=\frac{1}{8}\sqrt{2\cdot 5}(|6,6>+|6,-6>)-\frac{1}{8}\sqrt{2\cdot 11}(|6,2>+|6,-2>)\]
\[|\psi_{7}>=\frac{1}{12}\sqrt{3\cdot 11}(|7,6>-|7,-6>)+\frac{1}{12}\sqrt{3\cdot 13}(|7,2>-|7,-2>)\]
である。
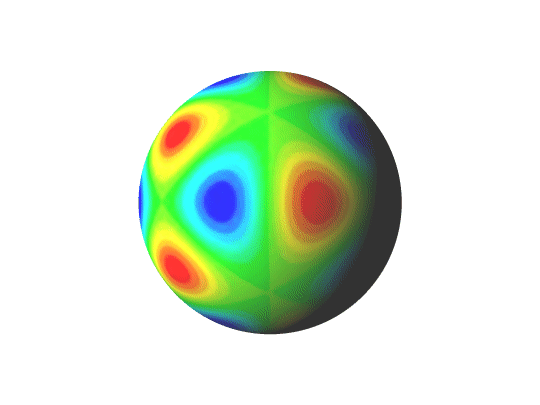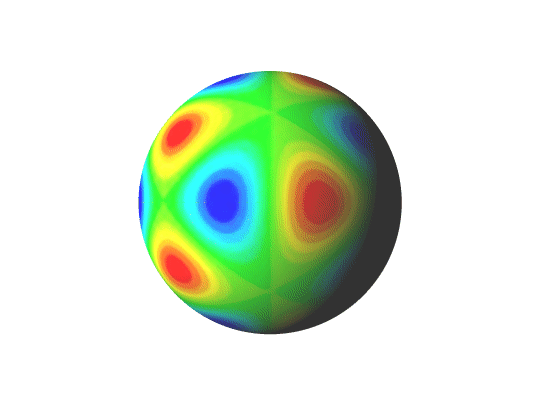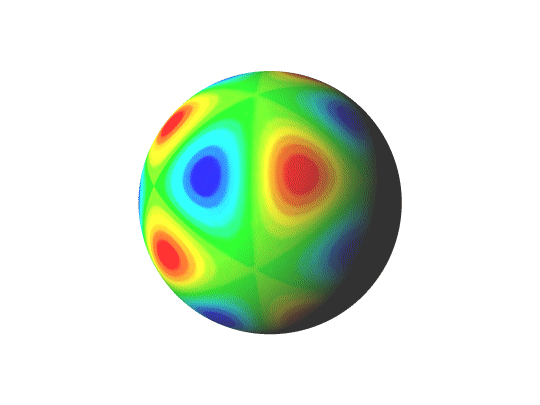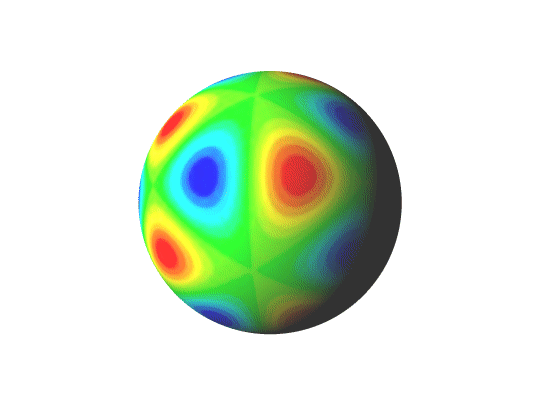

\(2\)つの\(L=3\)の合成系から生成された\(L=6\)の基底は\(z\)軸回りの\(1/4\)回転についても不変で、正八面体の対称性ももつので、
\[|\psi_{6,o}>=\frac{1}{4}\sqrt{7}(|6,4>+|6,-4>)-\frac{1}{4}\sqrt{2}|6,0>\]
と表し、\(L=3\)と\(L=4\)の合成系から生成された\(L=6\)の基底は、
\[|\psi_{6,t}>=\frac{1}{8}\sqrt{2\cdot 5}(|6,6>+|6,-6>)-\frac{1}{8}\sqrt{2\cdot 11}(|6,2>+|6,-2>)\]
と表して区別する。\(|\psi_{6,o}>\)は\(IC_{4}\)について不変なので、\(A_{1}\)表現の基底であるが、\(|\psi_{6,t}>\)は\(z\)軸回りの\(1/4\)回転について符号を変えるので、\(IC_{4}\)についても符号を変え、\(A_{2}\)表現の基底である。